- The ratios of the sides of a right triangle are completely determined by its angles.
- Because there are three sides of a triangle means that there are also three possible ratios of the lengths of a triangle's sides.
- These three ratios are the sine, cosine, and tangent trigonometric functions.
Do you know what two angles living inside the same right triangle said to each other? The first angle goes, “Hey Thelma (or is it Theta?), I don’t mean to go off on a tangent here, but what’s your sine?” To which the second angle replies, “Phil (or is it Phi?), I don’t know why you even bother to ask, my sine is obviously the same as your cosine!”
Okay, so maybe that’s not the best joke in the world, but once you understand sines and cosines, it is kind of funny. Of course, that means that if you don’t know the difference between a sine and a cosine, you’re currently left out in the metaphorical cold.
Clearly we can’t let that happen—and we won’t! Because today we’re going to learn all about sines, cosines, and tangents.
Recap: trigonometry and triangles
When we talked about the world of trigonometry, we learned that the part of math called trigonometry deals with triangles. And, in particular, it’s the part of math that deals with figuring out the relationship between the three sides and the three angles that make up every triangle.
Of particular interest to us is the special type of triangles known as right triangles. Every right triangle has one 90-degree angle (like the corner of a square or rectangle), and two angles that each range between anything larger than 0 degrees and smaller than 90 degrees (with, as we’ll talk about in the future, the sum of all 3 angles being 180 degrees).
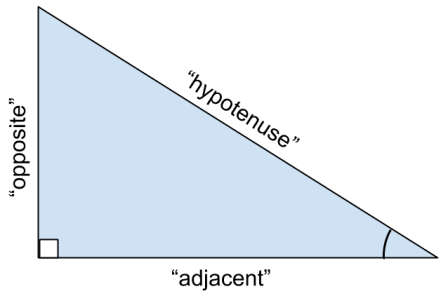
For our discussion of sine, cosine, and tangent (which, don’t worry, are not as complicated as they sound), it’s important that we have a way of labeling the sides of right triangles.
As we learned last time, the longest side of a triangle is known as its “hypotenuse.” The side opposite the angle we’re looking at is known as the “opposite” side (logically). And the side adjacent to the angle we’re looking at (the one that isn’t the hypotenuse) is known as the “adjacent” side.
Sine, cosine, and tangent
With all of these preliminaries now happily splashing around inside our growing pool of mathematical knowledge, we’re finally ready to tackle the meaning of sine, cosine, and tangent. Here’s the key idea:
The ratios of the sides of a right triangle are completely determined by its angles.
The ratios of the sides of a right triangle are completely determined by its angles.
In other words, the value you get when you divide the lengths of any two sides of a right triangle—let’s say the length of the side opposite one of its angles divided by its hypotenuse—is entirely set in stone as soon as the angles are set in stone.
Why? Well, if the angles are fixed, making the triangle bigger or smaller has no impact on the relative lengths of its sides. But changing the triangle’s angles, even a tiny bit, does! If you need some convincing, try drawing a few triangles of your own and you’ll see that it is indeed true.
Now, the fact that there are three sides of a triangle means that there are also three possible ratios of the lengths of a triangle’s sides. And, as you might have already guessed, these three ratios are none other than the famous sine, cosine, and tangent trigonometric functions.
What is SOH-CAH-TOA?
The sine of one of the angles of a right triangle (often abbreviated “sin”) is the ratio of the length of the side of the triangle opposite the angle to the length of the triangle’s hypotenuse. The cosine (often abbreviated “cos”) is the ratio of the length of the side adjacent to the angle to the length of the hypotenuse. And the tangent (often abbreviated “tan”) is the ratio of the length of the side opposite the angle to the length of the side adjacent.
Since this is kind of a mouthful and a little hard to remember, kind folks over the centuries have come up with a handy mnemonic to help you (and countless generations of kids in school) out. All you have to remember is SOH-CAH-TOA. In other words:
- SOH → sin = “opposite” / “hypotenuse”
- CAH → cos = “adjacent” / “hypotenuse”
- TOA → tan = “opposite” / “adjacent”
Real world trigonometry
You might be wondering how trigonometry applies to real life. How will you use sine, cosine, and tangent outside the classroom, and why is it relevant?
There are a few career paths that lead to constant use of these equations. For example, lets say you’re a sound engineer working on the production of a hit artist’s new album. You know that sound travels in waves, and engineers can manipulate these waves (measured by and applying trigonometry) to create different computer-generated sounds.
What if you’re an architect who needs to know the height of an existing building in a neighborhood you’re assigned? You can use the distance you are from the building and the angle of elevation to detemine the height. You can even use trig to figure out the angles the sun will shine into a building or room.
Construction workers also use sine, cosine, and tangent in this way. They need to measure the sizes of lots, roof angles, heights of walls and widths of flooring, and even more.
Crime scene investigators use trigonometry to determine the angles of bullet paths, the cause of an accident, or the direction of a fallen object.
What about on a crime scene? Investigators can use trigonometry to determine angles of bullet paths, the cause of an accident, or the direction of a fallen object.
NASA uses sine, cosine, and tangent. Its physicists and astronauts often use robotic arms to complete assignments in space and use trigonometry to determine where and how to move the arm to complete their task.
Thinking about studying marine biology? In this career, sine, cosine, and tangent are sometimes used to determine the size of large sea creatures from a distance, and also to calculate light levels at certain depths to see how they affect photosynthesis.
There are dozens of careers that use trigonometry in their daily tasks. So, you can stop saying things like, “I’ll never use trigonometry in the real world.”
What’s next?
While all of this talk about the angles and sides of right triangles and their correspondence to one another through the beauty and magnificence of trigonometry is indeed lovely, it might leave you wondering a bit about the “Why?” “What?” and “When?” of it all. By which I mean:
- Why exactly is this useful in the real world?
- What are the sin, cos, and tan buttons on my calculator for? (And how do they work?)
- When might I ever actually want to calculate the sine or cosine something?
Those, obviously, are all very important (and very reasonable) questions to ask. And they’re also very important questions to answer. Which is precisely the task we will begin to undertake next time.